SPECIAL EDITION
Hypotheses
The Quantum Nature of Biological Intelligence
Manzalini A1, Galeazzi B1
1Project on Foundations of Consciousness and Physics (PFCP), Integrative Medicine Clinic, Via G. Volpato, 45, ZIP-CODE: 36061 Bassano del Grappa, VI, Italy.
Authors’ emails:
Manzalini A (manzalini@gmail.com); Galeazzi B (bruno@galeazzi.info)
Keywords: quantum field theory, raman spectroscopy, biological intelligence, Nambu Goldstone bosons, quantum coherence.
Received: July 28, 2021
Revised: February 7, 2022
Accepted: March 4, 2022
Published: April 25, 2022
Highlights
1. The principles of quantum field theory/quantum electrodynamics (QFT/QED) and Gauge frameworks explain multi-level coherence in living organisms, in terms of balanced competition between Gauge and Nambu Goldstone (NG) bosons, as emerging from symmetry breakings (SB);
2. Biological Intelligence (BI) is an expression of connectedness, multi-level coherence and reactive adaptability in living organisms;
3. The presence of NG boson condensations, responsible for order and coherence in living matter, can be studied through Raman spectroscopy.
Abstract
Living organisms can be considered open systems, operating far from thermodynamic equilibrium – and creating, storing and exchanging energy, matter, and information with the environment. Overall, through these capabilities, living organisms pursue continuous self-adaptation to environmental changes, which is the expression of Biological Intelligence (BI). This paper argues that self-adaptation, and, in general, BI, is based on symmetry breaking (SB) phenomena that are well explained by an extension of the principles of quantum field theory/quantum electrodynamics (QFT/QED) and Gauge frameworks. SBs would be responsible for the emergence of multi-level coherence in living organisms, in terms of balanced competition between Gauge and Nambu Goldstone (NG) bosons. This balanced competition of bosonic fields, across all organisms, would allow the coupling with the environment up to the quantum level. Leveraging on the fact that more than 70% of the body is made up of water, the paper proposes a practical method, based on Raman spectra measures in water, for detecting NG boson condensations responsible for ordering information, coherence and memory storage in living matter.
Introduction
Living organisms can be considered open systems exchanging energy, matter, and information with the environment – and operating far from thermodynamic equilibrium. This high-level model is compliant with the definition of dissipative structures proposed by Nobel Prize winner Ilya Prigogine. His seminal work has been expanded by several authors, over the last decades, to cover four main types of dissipative structures: multi-stable states, temporal dissipative structures, spatial dissipative structures, and spatiotemporal structures in the form of propagating waves (Goldbeter, 2018).
Overall, these exchanges of energy, matter, and information are carried out through the non-linear interactions of billions of different components, up to the quantum scale. These exchanges are mediated by wave-like vibrations including, for example, mechanical, electromagnetic, nuclear and thermal oscillations. Even the vacuum, according to the Quantum Field Theory (QFT), is a ground level of energy, an arena of quantum fluctuations (e.g., virtual particles). All these vibrations and oscillations create, through complex interference patterns, a continuum of quantum fields capable of shaping matter’s structure, coherence levels and functionalities (Umezawa, 1993; Blasone et al. 2011).
As a matter of fact, attempts to apply quantum physics to living organisms began soon after the emergence of Quantum Mechanics (QM). One of the first remarkable examples of this occurred in 1944 when E. Schrodinger wrote his book, “What is life?” Pioneering research by H. Fröhlich started to demonstrate that the concept of quantum coherence is an inherent property of living cells, used for long-range interactions such as synchronization of cell division processes. This avenue (Ricciardi and Umezawa, 1967; Fröhlich, 1968; Del Giudice, 1983, 1988) has been confirmed also by recent advances in quantum biology that demonstrate that coherence is one of the key quantum phenomena supporting life dynamics (Ball, 2011; Salari et al. 2011). Coherent phenomena are well explained by QFT, a well-established theoretical framework within quantum physics. Moreover, quantum electrodynamics (QED), a declination of QFT dealing with the interactions between electromagnetic fields and matter, provides theoretical models and experimental frameworks that demonstrate how electromagnetic fields and potentials play a fundamental role in the emergence and evolution of coherent structures (Preparata, 1995), even in living organisms (Del Giudice, 1983, 1986).
This paper argues that the overall state of a living organism is an expression of a multi-level coherence that is created and regulated by quantum informational fields that, in turn, steer biochemical reactions. In a more general sense, it argues that the quantum nature of Biological Intelligence (BI), which is defined as an organism’s capacity to pursue active adaptation, is to maintain inner coherence while dynamically interacting with the environment.
In sum, the main contributions of the paper intend to:
1. Extend the principles of QFT/QED and Gauge frameworks for modelling multi-level coherence in living organisms, in terms of balanced competition between Gauge and NG bosons, as emerging from SBs;
2. Describe BI as an expression of the connectedness, multi-level coherence and reactive adaptability of an organism;
3. Propose a practical method, based on Raman spectra, for detecting NG boson condensations responsible for order and coherence in living matter.
Structure of the Paper
Section 1 describes the basic principles of QFT/QED and Gauge theory. Section 2 highlights the importance of coherence in living organisms. Section 3 defines the concept of BI in terms of the informational quantum field. Section 4 describes certain biological dynamics merging physicians’ and physicists’ perspectives. Section 5 proposes a practical method, based on Raman spectra, for detecting said quantum informational fields in living organisms. Conclusions and perspectives on future research close the paper.
Discussion
1. The Basic Elements of QFT and Gauge Theory
Presently, QFT is recognized as a fundamental theory in quantum physics, standing as the basis of the Standard Model. QFT provides an information-theoretical reinterpretation of physical reality confirmed by several experimental validations (Peskin, 2018).
One remarkable aspect of QFT is that quantum entities, such as particles, are modelled as excited states of underlying fields. The concept of field is rather intuitively well known: we recall that a field is defined as a property of the system under study that could be represented by a scalar, a vector, or a complex number, etc. Examples of force fields are the gravitational, the electromagnetic, and the strong and weak nuclear fields; examples of matter fields are the electron, neutrino, and up quark and down quark.
Therefore, QFT is overcoming the limitations of Quantum Mechanics (QM) by providing an intuitive explanation for the particle-wave dualism and other quantum phenomena such as entanglement. Put simply, quantum particles could be pictured as ripples of a corresponding underlying field.
Nevertheless, sometimes the difference between QM and QFT is not fully understood. Another key difference that is important to this paper is that, in QFT, every quantum system is considered an “open” system, forever interacting with background fluctuations. This has remarkable consequences – such as the fact that the Hamiltonian vision of every quantum system (even living organisms included) always comprises both the quantum system and its thermal bath of surrounding environment (Umezawa, 1993; Vitiello, 1995).
SB is another physical phenomenon that is well described by QFT (Umezawa, 1993). SB concerns the transition of a quantum system from a symmetric state to another at lower symmetry. Lower symmetry means higher order and, therefore, lower entropy. As described in the next sections, for example, a quantum system can reach certain levels of coherence (i.e., order) through SBs.
We need to distinguish two types of SBs: explicit SB and spontaneous SB. Explicit SB implies that a perturbation is determining a change of the basic dynamics of the system. In spontaneous SB, the dynamics are not changed – and the perturbation, which is weak, acts only to trigger the SB. In this case, the strength or the intensity of the perturbation isn’t important, but, rather, its phase (Blasone et al. 2011): in other words, in order for it to happen, the SB should be somehow in phase with the system. Therefore, a multitude of perturbing inputs will not affect a coherent system unless one or more have the same phase of the system. In particular, the Nambu-Goldstone theorem proves that SB implies the presence of NG quanta: this has been validated by solid experimental evidence both in high-energy and condensed matter physics. Phonons in crystals, magnons in ferromagnets, etc, are NG quanta originated by SBs being responsible for the space-time ordering observed in such systems.
NG bosons are massless and collective vibrational modes coding ordering information (Ricciardi, Umezawa, 1967; Vitiello, 1995). They do not mediate force interactions; rather, they represent the ordered modalities of such interactions. NG bosons disappear when the coherent state of matter vanishes (e.g., phonons in crystals). NG bosons are quasi-particles and should not be confused with Gauge bosons.
Let’s open a brief discussion on the Gauge theory. Within the context of the QFT and the Standard Model, the Gauge theory describes interactions and energy exchanges between quantum particles and their associated wave fields (O’Raifeartaigh, Straumann 2000). In the Gauge theory the Lagrangian (difference of the kinetic and the potential energy) of a system is kept invariant under continuous local symmetry transformations (called local gauge transformation), thanks to the presence of the gauge fields.
These fields are a sort of compensatory field capable of maintaining symmetry: examples of Gauge fields are the electromagnetic field, the weak gauge field in elementary particle physics, and the color gauge fields in quarks dynamics.
What happens with the SB, i.e., with emergent NG bosons prescribing a coherence state to the system’s components? Global phase symmetry is broken, so a compensation is expected between the long-range ordering NG correlation (as from the SB) and the long-range interactions mediated by the Gauge fields.
In fact, propagation of the Gauge fields changes in the different ordered configurations due to the different ways in which NG and Gauge bosons interact. This is a sort of balanced competition between the long-range ordering correlations of NG and Gauge bosons. The balance is reached depending on the boundary conditions and on the relative strengths of the Gauge and NG fields. Both forces and ordering correlations are actively present in all living beings, and their balance is manifest in the multi-level coherence of living organisms.
Even if further technical details are outside the scope of this paper, the above principles are fundamental assumptions on which the thesis of this work has been developed: the interplay between Gauge and NG bosons (as emerging from SB) in living matter plays a key role in producing biological features and functions (Del Giudice, 1986). Also, the characteristic behaviors (e.g., coherence at room temperature), shapes (e.g., fractals) and singular properties (e.g., chirality) present in living matter could be explained by invoking the principles of QFT.
Eventually, the more advanced steps of this work will find inspiration in the words of H. Pattee: “I am convinced that the problem of the origin of life cannot even be formulated without a better understanding of how molecules can function symbolically, that is, as records, codes, and signals” and “life is matter controlled by symbolic information” (Pattee, 1969). Furthermore, we argue that said quantum phenomena in living organisms correspond to symbolic abstractions, images or even more archetypes as described by C.G. Jung. This is obviously more complicated to demonstrate, but it could build a bridge between Mind and Matter (Seager, 2009), as dreamt by C.G. Jung and W. Pauli (Nobel Prize in Physics).
2. Coherence in Living Organisms
Coherence phenomena have quite general characteristics that can be found in the physics of elementary particles, condensed matter, cosmology and biological systems.
Several studies in the last decades (Ricciardi, Umezawa, 1967; Fröhlich, 1968; Del Giudice, 1983, 1988; Vitiello, 1995, 2001) and recent advancements of quantum biology demonstrate that coherence, as a state of order of matter coupled with electromagnetic fields, is one of the key quantum phenomena at the basis of life (Al-Kahalili, Mc Fadden, 2015).
As a matter of fact, coherence enables long-range correlations between the components of a system. This, in principle, allows the highly efficient performances of living processes. For instance, in a coherent system, every process of molecular recognition based on a simple “chaotic/diffusive lock-and-key” principle is replaced by long-range electromagnetic resonance (Cosic, 1994).
Fifty years ago, H. Fröhlich (1968) created the concept of quantum coherence as an inherent property of living cells in long-range interactions such as the synchronization of cell division processes.
Another example is reported by Lloyd (2011): he argues that the very high efficiency of photosynthesis is due to coherent excitons (electron–hole pairs) capable of harvesting light quanta of energy and of funnelling them at specific sites in the shortest possible time interval. Moreover, the dynamic emergence of coherent, spatially distributed oscillations is also recognized as a key characteristic of human brain connectome (Vitiello, 1995, 2001; Freeman, Vitiello, 2006; Atasoy et al. 2016).
We recall that a coherent system is characterized by a single rhythmic oscillation, let’s say, a unique phase, φ. This phase is indeed the expression of the fact that all the components of the quantum system are correlated over long distances. This has important consequences: for example, the states of coherent systems can be characterized, by looking at their phase, φ, as a single macroscopic observable, a so-called order parameter of a SB. Therefore, coherence allows moving the description of a system from the quantum-scale up to the macroscopic one (Umezawa, 1993; Blasone et al. 2011; Messori, 2019).
The Informational Role of NG Bosons in Coherence
According to QFT, the dynamics that regulate the behavior of elementary components in a physical system can generate the formation of coherent structures with a large extension compared with the dimensions of the components. Mathematical analysis and experimental confirmations show that this coherence emerges from a break of symmetry: a correlation wave is generated whose effect is to put in phase (phase locking) the elementary components (Del Giudice, 1988).
The quanta associated with it are NG bosons. As mentioned, NG bosons have zero mass (in absence of edge effects) and they do not contribute to the energy of the ground state: the accumulation, or condensation of NG bosons in the vacuum state generates a state of minimum energy, called condensed state (Del Giudice et al. 1983, 1988). Such a condensed state is therefore stable (minimum energy state). NG bosons can be observed with diffusion techniques (i.e., using them as targets of particles, e.g. neutrons); the energy spectrum is studied by exciting them, deforming the ordered structure (e.g. crystal lattice) by external tensions, thermal jumps, etc.
Condensation doesn’t take place in the presence of “edges,” that is, when the volume of the system cannot be considered infinite. A non-zero “effective” mass then limits the capacity of the NG quanta to propagate over the whole system and unrelated or disordered regions are generated (Del Giudice et al., 1985).
Therefore, the system appears as a mix of two phases, one coherent (ordered) made up of coherence domains, the other non-coherent (disordered or symmetrical) interposed between the domains (interstitial). Under such conditions, the electromagnetic field and the field describing the elementary components couple in a coherent way (phase locking). Furthermore, the electromagnetic field acquires a mass by remaining confined in the region of the domain (i.e., the Anderson-Higgs-Kibble mechanism; Del Giudice et al. 1986). This phenomenon has very interesting consequences in many contexts, including phenomena observed in biology that are characterized by high efficiency, selectivity and stability.
Another important aspect that should be mentioned is that, in QFT, the uncertainty principle connects the phase of a system and the number of elementary components comprising it, according to this formula:
ΔN Δφ ≥ ½
where N is the number of components of the system.
As coherence means a well-established phase φ, the optimal requirement for a living organism should be a vanishing of Δφ, which in turns implies a ΔN as large as possible. This means that being a healthy organism compels it to extend a dialogue with a large part of the external world, which becomes its own double (Vitiello, 2001).
In other words, quality of life depends on the capability of an organism to maintain a sharp definition of the phase. In this respect, the connectedness of the organism is essential for constructing and maintaining a well-defined phase during the unpredictable events of life.
These assumptions bring us to develop a mathematical model in which organelles, cells, tissues, organs, organs systems, up to a whole organism, are characterized by their own specific wave-functions, whose phases are perfectly orchestrated in a multi-level coherence oneness (Dal Lin et al. 2020). Should the organism, or some of its components, lose its rhythm or phase, then the oneness of the multi-level coherence is broken, and disease emerges. The healthy resilience of a living organism depends on the ability to keep the natural oneness of this multi-level coherence across the body in its environment.
A very similar conclusion has been argued by H. R. Maturana (1995) in the following terms: “Due to this systemic relation between living system and medium, the structural dynamics of a living system are always, while it lives, spontaneously in adequate structural coincidence with the medium for the realization of its living. When such coincidence disappears, the living system dies.”
The Free-Energy Minimization Principle, Fractal Self-Similarity
The free-energy minimization principle has been already proposed for modelling biological nervous systems (Vitiello, 1995, 2001; Sengupta et al. 2016) and the brain (Vitiello, 1995, 2001; Tozzi and Peters, 2016). In these cases, the minimization of the variational free energy describes how a biological system changes its internal states so that it becomes the most suitable under the influence of external states of the environment. In doing so, the system is implicitly learning a generative model of the environment: the better the model is in making predictions, the lower the variational free energy.
In Friston’s papers (2008 and 2010), the free energy principle has been proven valid for describing several aspects of functional brain architectures. Minimizing variational free energy ensures that the brain becomes a good model of its environment: this has again a striking correspondence with the concept of “double” described in terms of QFT (Vitiello, 2001).
The interactions between a system and its environment determine the doubling of the collective modes of the overall ensemble, i.e., the “system-environment,” as the energy flux balance is preserved. In this dissipative model of the brain (Vitiello, 1995, 2001, 2004), an external stimulus is responsible for spontaneous SB thus generating NG bosons, which bring a new level of coherence to the organism.
It is also known that fractal self-similarity can be expressed in terms of squeezed coherent state and vice versa: squeezed coherent state and fractal self-similarity are mathematically identical (Vitiello, 2012).
Therefore, we can also argue that, in a living organism, squeezed coherent state and fractal self-similarity are characteristics of organelles, cells, tissues, organs, organs systems, up to a whole organism, leading to a multi-level coherence oneness.
In the human body there is evidence of many structures that appear to be fractals: the networks of blood vessels, the networks of neurons, the ramifications of heart muscles, and the bronchial tree.
Should the organism, or some component, lose its natural coherence level, then the oneness of the multi-level coherence is broken, and a disease emerges, driving the system away from the minimal free energy principle (Dal Lin et al. 2020).
3. Biological Intelligence (BI)
Intelligence has been defined in many ways, but, in general, it can be described as a living organism’s ability to sense, perceive the environment, extract and develop related information in order to create the knowledge that is necessary for actuating self-adaptive behavior. Therefore, as argued by Plotkin (1994), intelligence can be defined as the capability, often species-specific in manifestation, which generates adaptive behavior.
This definition of intelligence is perfectly in line with the so-called principle of minimal free energy (see next section).
In this paper, we named BI the capability of an organism to pursue an active adaptation, to maintain inner coherence, while dynamically interacting with the environment.
The proposed definition of BI is analogous to the modern definition of health, as emerged from the “global conversation” coordinated by Jadad and O’Grady: the ability to adapt and self-manage in the face of social, physical, and emotional challenges (Jadad and O’Grady 2008; Godlee 2011).
BI is an expression of the levels of connectedness and coherence of the organism. The former (connectedness) impacts quality of life, as it is essential for constructing and maintaining the capability of resonating with the environment: in other words, being a healthy organism compels us to dialogue with a large part of the external world. The latter (coherence) is an expression of the healthy state of an organism.
Bridging the concept of coherence from physics to physiology, coherence occurs when two or more of the body’s oscillatory systems, such as respiration and heart rhythms, oscillate at the same frequency (Dal Lin et al. 2020). In systems that exhibit sine-wave-like oscillations, the more stable the frequency, amplitude and shape, the higher the degree of coherence.
When coherence is increased in a system that is coupled to other systems, it can pull the other systems into increased synchronization and more efficient functioning (McCraty et al. 2009). Frequency pulling and entrainment can easily be seen between the heart, respiratory and blood pressure rhythms, as well as between very-low-frequency brain rhythms, craniosacral rhythms and electrical potentials measured across the skin (Bigger et al. 1992; McCraty, 2010).
How does BI operate while trying to achieve an active adaptation?
In principle, when following Artificial Intelligence modes, we would be prone to consider BI operating through logical mechanisms, like right/wrong, yes/no, on/off, according to a binary code. This is how the rational mind operates: the choice is between two opposite possibilities; no third option is available.
When we consider clinical practice, the person manifesting a disease is stuck in a conflict between two possibilities. In the following paragraphs, we’ll see that the modalities of individual expression of physical disease are related to a person’s particular emotional/psychological suffering. Therefore, psychological suffering is usually present, and is related to dichotomous unsolvable situations. An example can help clarify the concept.
The patient’s conjugal relationship is facing a great crisis; he/she doesn’t want to break up because of moral duties, yet is incapable of changing the relationship for the better. Staying or going away are both unacceptable solutions, but the person can’t see any other option. It is not the conjugal conflict or the conflict inside the patient; being blocked inside the conflict is the main source of suffering.
It’s common, in daily life, to be confronted with difficulties that require an individual response, but the subtleties of the situation and the individual interpretative framework do not allow an easy, dichotomous, right-or-wrong solution. Any daily situation is a stimulus to the individual system: it excites the senses that incorporate the incoming signal; then the signal is interpreted by the system, acquiring a meaning for the system and, according to the meaning, a system’s response is manifest. In the interpretation process, two different mechanisms are at work.
The most obvious is the logical mechanism that answers the question, “why?”; a chronological and logical explanation followed by a yes/no, right/wrong set. The second interpretation mechanism works through analogy and answers the question “how is it?” or “how does it feel?”
The analogy questions dig into the world of sensations and images of the patient; therefore, an almost innocuous signal could be interpreted as if it were a tremendous life threat, because that specific stimulus finds memories and psychological images that make it feel so disruptive.
In the therapeutic setting, the internal conflict of the patient isn’t overcome by forcing choice of one of the two polarities, right/wrong or true/false, but by acquiring the ability to understand how the situation is interpreted through analogical thinking and introducing a solution that integrates the set of interpretations, thus bringing the system to a higher level of psychological coherence, overcoming the dichotomy right/wrong, true/false and producing, out of the two dichotomous options, a third coherently integrated solution.
It’s worth underlining that the coherently integrated solution, or adaptive solution that respects the free-energy minimization principle, doesn’t follow the fuzzy logic explanation, as it’s not only an adaptive answer placed in between two dichotomous polarities, but it’s a new solution that emerges integrating different mindsets of variables, including interpretational analogies produced by the individual (Penrose, 1994; Freeman, Vitiello, 2006). Adaptive mechanisms appear therefore as creative processes that, accordingly to the free-energy minimization principle, produce effective solutions, in an integrative process that resembles, in the mind, the collapse of superposition states elsewhere observed, e.g., the highly efficient photosynthesis process, based on superposition states in the solar pigments compared to the on/off mechanism of artificial silica layers in photovoltaic panels (Thyrhaug et al., 2018).
This reasoning has striking similarities with the Path Integral model, which addresses the problem of determining the trajectory of a particle-like object from A to B. Richard Feynman’s suggestion was that, in moving from A to B, the particle-like object will take every possible trajectory: forward and backward in time, zig-zagging, looping, whatever. To get the amplitude probabilities, Feynman argued that each trajectory contributes a complex factor, where S (time x energy) is the action describing that trajectory. All contributions should be added up to get the overall amplitude (i.e., the sum over histories). Each path is weighted by a phase factor, and there’s some degree of cancellation between the contributions from different paths. Certain trajectories will possess essentially random phases and will cancel each other, but other trajectories that are sufficiently close to the stationary value (predicted by Lagrange in classical systems) would have similar actions and thus similar phases, giving a strong in-phase contribution. Therefore, the sum is dominated by the stationary result, apart from certain quantum fluctuations. This is still valid and extremely interesting when applied to the transition of a living being from a state A (e.g., healthy) to a state B (e.g., illness) or vice versa.
The characteristics of BI, as conceived above, are well displayed in the reactive dynamics that clinical psychologists and physicians observe in their patients. Psychological defense mechanisms are a clear example of the attempt of BI to reduce free energy, notwithstanding the system’s unsuccessful attempt to react to the stimulus with fully adaptive behavior. The defense mechanism marginalizes the increment of free energy – avoiding, blocking or encapsulating the triggering stimulus, and thus partially optimizing the adaptive reaction and energy expenditure.
Also, as previously mentioned, at the physical level, the intrinsic adaptation of a living organism tries to minimize free energy and bring back the organism to its initial healthy condition, through some particular adaptive reactions. When an internal organ is affected, the system tries to bring the reactive process to the surface of the organism, thus reducing the impact on the organs more important for the economy of the system. Moreover, if adequately stimulated, the healing process proceeds chronologically backwards, firstly resolving the most recent symptoms and then the older ones, until the initial healthy condition is restored.
The healing process, when adequately triggered, is able to proceed backward eliciting older symptoms that aren’t manifest in the present disease picture, and to stimulate the BI reaction until the older symptom is completely recovered. Such a particular healing dynamic, observed through application of several psychological or physical therapeutic techniques, raises the question about the modality used by the organism to store past disease dynamics.
When observing the precise chronological backward path, we are tempted to present a daring hypothesis: any disturbance of the system stimulates a SB, followed by condensations of an NG boson, subsequently stored in the system according to the chronological sequence of SB events. The healing process brings back to actuality the SB experience condensed in the NG bosons. At completion of the healing process, the system has recovered a higher level of symmetry, therefore capable of a wider set of adaptive responses to stimuli.
In QM, the focus is placed on the behavior of the single particles, while, in QFT, the field is fundamental. The field is the environment where the single particles appear as excited states; the dynamics of the field environment, and the intricacies of the quantum fields’ relationships, give rise to the observed reality.
Similarly, in biology, focus should change from the single individual organism towards a systemic approach in which the single organism is considered part of its environment (Vitiello, 1995, 2001, 2004). The behavioral and adaptive reactions of the organism are conditioned and can be understood by taking into account at least three different environments (fields) in which it is immersed and to which it is related: the genetic/familial environment; the physical external environment; and the internal psychological environment. Both the behavior and the adaptive patterns of reaction emerge from the interdependent relationship between the above-mentioned environments. Those environments can facilitate or hinder the organism’s adaptive reactions according to their specific characteristics. The genetic environment includes chromosomal material, epigenetic information and transgenerational behavior patterns. The external environment includes material substances, beneficial and noxious for the organism, and the several quantum fields to which the organism is related. The internal environment includes the psychological characteristics and memories that build up the structure of a human organism’s individuality. A detailed dissertation of the above characteristics is beyond the scope of this article, but it’s worth mentioning the analogies of a systemic approach from physicists’ and physicians’ point of view.
4. From Physicists to Physicians
At a fundamental level, life is quantum mechanical (Arndt et al. 2009; Huelga and Plenio, 2013; Marais et al. 2018; McFadden and Al-Khalili, 2018), although, through rough approximation, in biology, classical physics and biochemistry are used to describe the macroscopic phenomena observed in living systems.
Some of the greatest achievements in modern medicine, both in the diagnostic and the therapeutic fields, are obtained through extensive use of quantum technologies; nevertheless, the interpretative framework of biological dynamics is still rooted in the classical physics and biochemistry paradigm. Several observations in biological and clinical settings that cannot be fully explained by the current conceptual framework stimulate the need to widen the paradigm.
From a physician’s perspective, the theoretical achievements of modern physics offer explanatory clues for certain fundamental aspects of biological systems and human health.
In particular, we are going to examine the following aspects:
• Personal memory and transmission of transgenerational patterns and behaviors
• Physical signs and symptoms as metaphors. A metaphor is an imaginative way of describing something by referring to something else, which is the same in a particular way. In the next paragraphs we’ll explore this concept in more detail.
Personal Memory and Transmission of Transgenerational Patterns and Behavior
The seminal work of academic psychologist Anne Ancelin Schützenberger (1998) has elucidated the intriguing and unexpected consequences of personal experiences in the economy of transgenerational behavior of descendants. Unresolved psychological issues and traumas can remain stored in transgenerational “crypts,” and recursively manifest, over subsequent generations, through unconsciously reproduced behavior patterns that show productive characteristics around a central familial issue. When analyzed in the psychological framework, those recursive behavior patterns are like fractals, dynamically arranging themselves around an attractor represented by the central familial issue stored in the transgenerational crypt. The unconscious characteristics of these recursive events or behaviors are worth mentioning. Descendants are usually unaware of the events that hurt their ancestors and of the repetitive pattern over generations; only accurate psychological work is able to elucidate the pattern. Where is all that suffering and trauma, all those psychological wounds stored over generations? Can we identify genetic inheritance of those existential stigmata or are they stored and passed through generations somehow else?
This inquiry leads us to explore the concept of Jung’s collective unconscious: is that just a psychological tool useful in psychotherapeutic settings or does it have any foundations in physical reality?
Persinger (1987) has ascertained that temporal and regional variations in psychological processes have been associated with geological factors, thus showing the possibility of interconnectedness over long distances.
Jung’s hypothesis of a collective unconscious has been scrutinized in several ways over the last decades; the Global Coherence Initiative is probably the largest ongoing project investigating whether human emotions and consciousness interact with and encode information in planetary energetic fields, including the geomagnetic field, communicating information between people at a subconscious level; though exposed to some criticism, their work is still in progress (McCraty, 2012).
In our hypothesis, transgenerational memories could remain stored in massless NG boson condensations resulting from spontaneous SBs triggered by significant life events.
Physical Signs and Symptoms as Metaphors
In medical semiotics, signs and symptoms are indicators of bodily disorders from an objective and subjective perspective, respectively. The explanatory framework for their manifestation is fully acknowledged within the range of several biochemical molecular biology mechanisms, such as disruption of biochemical pathways; cascades of inflammatory cytokines; imbalance of the neurovegetative system; altered gut microbiota; mutations and alterations in gene expression; and many others. Over the last decades, signs and symptoms have been recognized as the result of the disruption of a complex network of organs and systems within the body that includes the psycho-neurological, endocrine and immune systems (PNEI) (Ader et al. 1995; Dal Lin et al. 2020).
Through interconnectedness, a therapeutic action on a side of the system is capable of modulating the whole network. As demonstrated by several experimental studies, modulation of the psychological environment produces physical modifications in signs, symptoms and biological parameters (Bottaccioli et al. 2014; Dal Lin et al. 2020).
The PNEI approach has reduced the distance between internal medicine and psychology. Freud is the founder of a psychological movement that has deeply investigated the correlations between mind and body, signs and symptoms and their symbolic significance. Franz Alexander (1950) described the role of the autonomous nervous system in the dynamics of impulse discharges aimed at maintaining the system’s balance, i.e., adaptive invariance under internal or external stimuli and the rising conflict.
According to Helene Flanders Dunbar, different personality traits develop different physical disease patterns, e.g., the hypertensive, the coronaropathic, the rheumatic personality. The Psychosomatic Paris School founded by Pierre Marty underlined patients’ difficulty to verbally express their suffering, alexithymia, thus transforming it into physical symptoms (Marty, 1980). According to Luis Chiozza (1998), the disease affecting a certain organ or apparatus can be understood considering the symbolic significance of that specific organ or apparatus in the context of a patient’s unconscious fantasies. Joyce McDougall (1989) identified the body as the stage in which the patient narrates his story; symptoms are metaphors of unresolved suffering and, through their physical manifestation, are symbolically representing the underlying travail.
As mentioned above, abundant psychological literature and a wealth of clinical evidence show the relevance of the physical symptoms as the place where suffering is expressed in the form of analogies, symbols and metaphors. The careful physician or psychologist can discriminate, in the words of the patient, those that are apparently insignificant, from a nosographic diagnosis point of view, but highly evocative of underlying psychological suffering that is frozen in images of the mind. In the theater of the body, a frightful stomach ache can be the manifestation of a trauma caused by a frightful experience whose verbal expression and resolution is missing and frozen in the psychological experience. The frightful experience, and how the patient interpreted it, remains unresolved and is described in the mental images that populate his or her mind. The frightful experience produces a spontaneous SB and, if it remains unsolved, acts like an attractor; the person has lost his or her full capacity of adapting to stimuli and is caught in recursive reaction patterns. Unresolved trauma keeps the organism in reactive dynamics that are far removed from optimal adaptation, and requires higher energy expenditure than the free minimum energy.
From a psychological point of view – and from the PNEI perspective – the transduction of mental images, trauma or inner conflicts into physical manifestation is taken for granted. Nevertheless, a gap exists between mental images and their transduction to physical symptoms, including alterations of biochemical pathways; genetic expression; imbalance of the neurovegetative or immune systems; and endocrine disturbances. In our hypothesis, the effects of spontaneous SBs, triggered by significant events and generating NG bosons, could act as mediators of the transduction of images from mind to symptoms (Freeman and Vitiello, 2006, 2016).
5. Experimental Validation Activities
This section describes the key research questions and the main assumptions adopted in the experimental activities aimed at validating the theory described in the paper.
A first key research question has been: how to detect the presence of NG bosons or, better, how to detect the effects of the interplay between Gauge and NG bosons in living matter?
In crystals, for example, phonons (which are a class of NG bosons) can be detected with neutron scattering techniques. Multi-spectral analysis can be used as well. As a matter of fact, every piece of matter in nature is pervaded by vibrations and oscillations: mechanical vibrations, electromagnetic oscillations, thermal, chemical-bonds vibrations, and quantum noise, etc. are some examples of this. Even the vacuum, according to QFT, is a ground level of energy, an arena of quantum fluctuations, e.g., in terms of fluctuating virtual particles (Ventura et al. 2017; Havelka et al. 2011; Pelling et al. 2004). The vibrations and oscillations of a system, in principle, contain certain levels of information, which are characteristic of the system.
Therefore, a first assumption has been that all these vibrations can be considered, in a way, expressions of the balanced competition between the long-range ordering correlations of NG and Gauge bosons. As already said, the balance is reached depending on the boundary conditions and on the relative strengths of the Gauge and NG fields.
A second assumption concerns focusing the attention on the vibrations of the hydrogen bonds, given their importance and pervasive presence in living matter. We assume that NG bosons, due to SBs, influence the vibrations of the hydrogen bonds. Hydrogen bonds are not only present in water, perhaps the most essential substance for life, but are also in deoxyribonucleic acid (DNA), between complementary base pairs to provide the structure with greater stability. There are three main types of atomic and molecular interactions: Coulomb, van der Waals, and chemical. Chemical interactions include several types of bonds involving atomic or molecular orbitals: covalent, ionic, donor-acceptor, hydrogen etc. The hydrogen bond energy is about an order of magnitude higher than the van der Waals energy, but it is the weakest among all types of bonds.
On the other hand, the length of the same hydrogen bond can vary over a broad area, and as well its spectroscopic parameters in vibrational spectra. The fractal behaviors of water in aqueous and biological systems and, in turn, of certain living structures, might be attributed to the vibrational characteristics of hydrogen bonds (Yagihara, 2019).
Over the last 50–70 years, numerous attempts have been made to model and measure the hydrogen bonds, in terms of their structural parameters and manifestation in vibrational (IR and Raman) spectra. There is a rich prior art on that: for instance, Kolesov (2021) provides an interesting overview about Raman spectroscopy (a detailed analysis of this prior art is outside the scope of this paper).
In Raman scattering, the wavelengths of the scattered light may decrease or increase; this is called Stokes Raman scattering or Anti-Stokes Raman scattering (which occurs in ground-state molecules), respectively. Anti-Stokes Raman scattering shows lesser intensity than Stokes Raman scattering; therefore, most Raman spectrometers use the Stokes Raman scattering. In Stokes Raman scattering, the wavelength of the incident light shifts due to the difference in the intrinsic vibrational energy of the molecule. Each molecule is characterized by specific vibrational energy, such that this frequency difference has its own value. Therefore, the composition or structure of a chemical can be identified through Raman scattered light.
Therefore, we consider the use of Raman spectroscopy as a means for detecting NG bosons influencing the vibrations of hydrogen bonds in water.
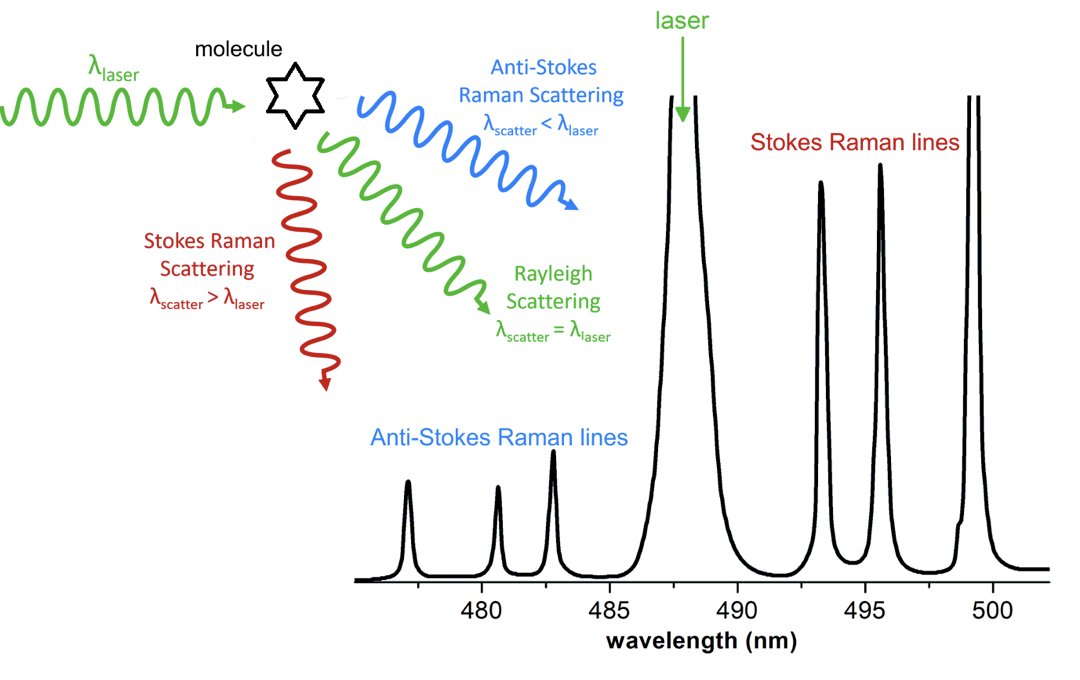
Figure 1: Raman scattering
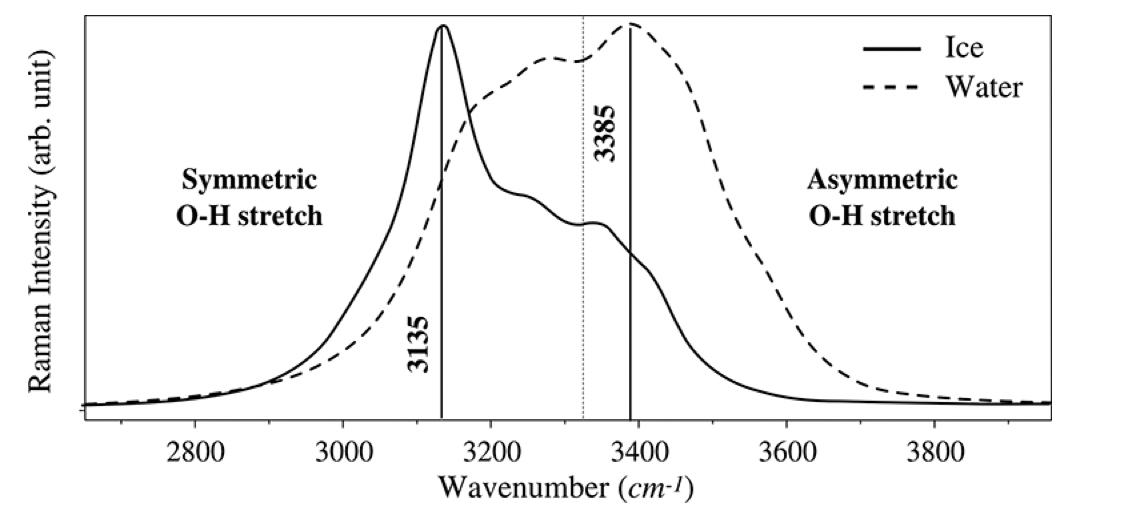
Figure 2: Raman spectra of water in the temperature range around the phase transition (between 3 and -3ºC) (Picture credits: Đuričković, 2016).
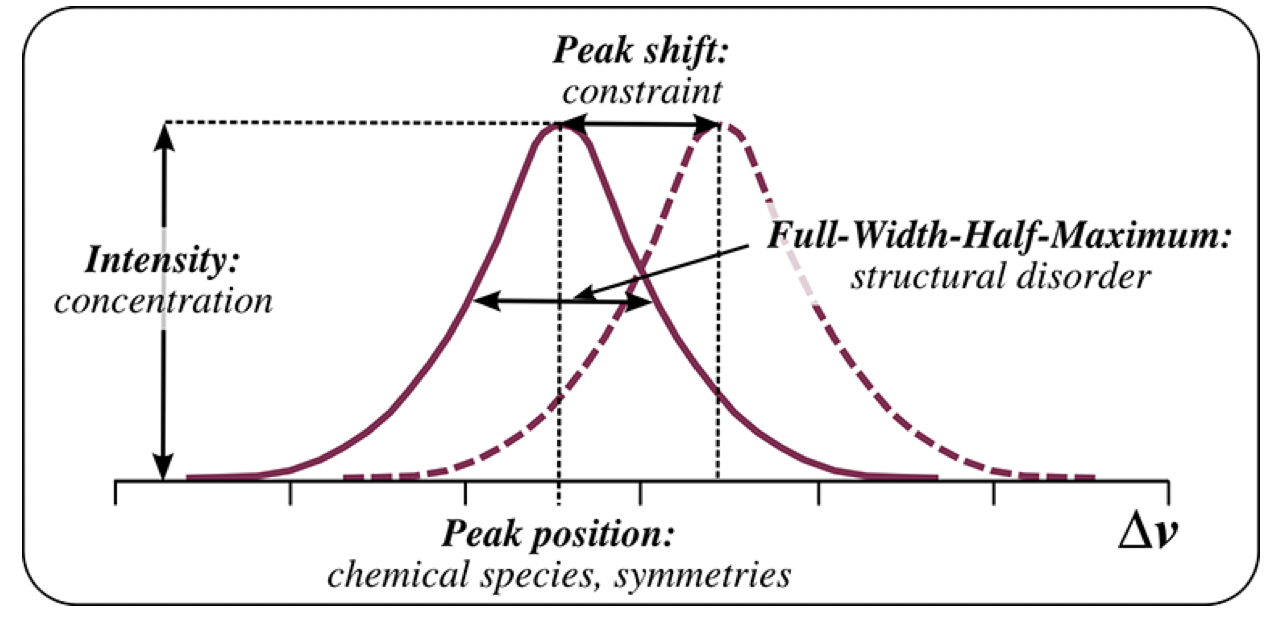
Figure 3: Information contained in Raman spectrum. (Picture credits: Đuričković, 2016).
For instance, Figure 2 shows the transition from water to ice; it can be considered as a SB generating NG boson: as a matter of fact, Raman spectroscopy of ice and water reveal different spectra (Đuričković, 2016).
In other words, it is argued that there is a direct link between the NG condensations and the Raman spectra of specific substances. In fact, a Raman spectrum can give us numerous qualitative and quantitative information about the sample: the position of the peak defined by its maximum corresponds to the vibration frequency of the chemical bond of the species; peak intensity is related to the corresponding chemical species concentration; the full width half maximum (FWHM) reflects the sample’s level of structural order: the lower the FWHM, the higher the local order. The peak shift towards the left and a smaller FWHM indicate the presence of NG boson condensations bringing more order to the substance (see Figure 3).
A mathematical model describing Raman spectrum relations with NG boson condensations and the Raman spectra is outside the scope of this paper, and will be presented later on by a companion paper.
Conclusions and Future Work
This paper starts by considering that living organisms can be considered open systems, operating far from thermodynamic equilibrium; developing, storing and exchanging energy, matter, and information with the environment. Overall, through these capabilities, living organisms pursue continuous self-adaptation to environmental changes, which is the expression of BI. The paper then argues that self-adaptation, and in general BI, is based on SB phenomena, well explained by an extension of the principles of QFT/QED and Gauge frameworks. In fact, SBs are responsible for the emergence of multi-level coherence in living organisms, in terms of balanced competition between Gauge and NG bosons, that allows coupling with the environment up to the quantum level. Leveraging on the fact that more than 70% of the body is made up of water, eventually the paper proposes a practical method, based on Raman spectra measures in water, for detecting NG boson condensations responsible for ordering information, coherence and memory storage in living matter.
Further understanding and scientific foundations for BI could be achieved through the interaction of multiple disciplines such as quantum physics, quantum biology, medicine and psychology, and we strongly encourage such efforts.
Conflict of Interest and Funding
There is no conflict of interest related to this article. The authors haven’t received any external funding.
Discussion With Reviewers (DWR)
Reviewer: In the paper, a sentence by H. Pattee is quoted: “Life is matter controlled by symbolic information.” Could you better explain to what extent it is possible to further investigate this concept of symbolic information in the proposed research framework?
Authors: An in-depth observation of clinical symptoms shows that every symptom possesses certain general characteristics, e.g. gastritis can include generic symptoms like burning, heaviness etc – but patients usually add more attributes to general symptoms, such as “scarring” burning, “unbearable” heaviness, or go directly to the analogy, such as “I feel burning as if it was a fire, or a volcano;” “I feel heaviness as if a mountain was parked on my stomach.” In these brief examples we see emotions, sensations, and analogies in the form of images. Usually, those images are discarded as colorful but useless information in the path towards a diagnosis. But if a physician further investigates these patients’ expressions, a portal can be opened beyond the rational, thus approaching an inner world inhabited by emotions, sensations, analogies, and metaphors, where images are the mediators of meaningful content. In the world of analogy, the “scarring” expressed with the burning appears in its original meaning – when patients recollect that some unresolved experience of the past had a “scarring” effect on them. The “fire as if a volcano” can be recognized by a patient as similar to what was experienced during times of anger or great turmoil, and so on.
So, on the clinical side, we find meaningful images, expressing deeper unresolved experiences, behind the appearance of physical signs or symptoms.
Next, we ask where these images come from. What is the origin of these images kept stored in the psychological world of the patient? In our hypothesis, the SB that occurs in the living system when confronted with external stimuli originates NG bosons that can remain stored in the system because of meaningful unresolved experiences. These NG bosons include the description of the event and the impact and reaction of the living system.
Reviewer: Some claim that Raman spectroscopy could be a valid means for detecting condensations of NG bosons. Why is it preferred to IR spectroscopy?
Authors: Raman spectroscopy allows detecting a change in the polarizability of molecules during the vibrations (phonons). Raman spectroscopy has several advantages over other vibrational spectroscopy techniques. These advantages occur because the Raman effect manifests itself in the light scattered off a sample, as opposed to the light absorbed by a sample. Therefore, Raman spectroscopy is insensitive to aqueous absorption bands, and requires little effort for sample preparation. Information about vibrational transitions is obtained by shining monochromatic visible radiation (laser) on the sample: a momentary distortion of the electrons in a bond of a molecule creates an induced dipole that is temporarily polarized; when the bond returns to its normal state, radiation is reemitted as Raman scatter. It should be mentioned that, often, IR and Raman spectra provide complementary information about many of the vibrations of molecular species. Eventually, Raman spectra are less complex than IR spectra.
Reviewer: Is it possible to use Raman spectroscopy to characterize the state of coherence of biological water or cells, tissues, etc? If yes, for example, could it be used for cancer diagnosis and prognosis?
Authors: Raman spectroscopic research in biology has been attracting more and more attention. In fact, the physicochemical properties of biomolecules could ultimately be interpretable in terms of their vibrational behaviors, as a sort of fingerprint left by their chemical structures and biological phenomena. As such, Raman spectroscopy appears to be a valuable method to characterize the state of coherence of biological water or cells, tissues, etc, and maybe also for supporting diagnosis and prognosis.
Reviewer: Would it be possible to get a better explanation of how your model could describe chirality in biological systems?
Authors: Chirality is the property of non-superimposable forms that are mirror images of one another (e.g., left, and right hands). For example, in the case of a chiral molecule, the two forms, called enantiomers, have identical physical and chemical properties, but the way each interacts with other chiral molecules may be different. Biological molecules show single chirality as they appear exclusively as left-handed amino acids and right-handed sugar. Moreover, the DNA double helix in its standard form always twists like a right-handed screw. The cause originating the imbalance between left and right enantiomeric biological molecules is still under study. One of the candidate theories is based on the idea of parity violation in nuclear weak interactions, involved in nuclear decay. In fact, weak nuclear force is the only force having a handedness preference (i.e., parity violation): electrons created in the subatomic process known as β decay are always left-handed (spin is always opposite in direction to the electron’s motion). Left-handed electrons have been found to destroy certain organic molecules faster than their mirror versions, which could have led to a SB in the balance between left and right enantiomeric biological molecules (Gibney, 2014).
Reference for Discussion with Reviewers
Gibney E (2014). Force of nature gave life its asymmetry. Nature (2014) DOI: 10.1038/nature.2014.15995.
References
Ader R, Cohen N, Felten D (1995). Psychoneuroimmunology: interactions between the nervous system and the immune system. The Lancet 345: 8942, 99-103
Alexander F (1950). Psychosomatic medicine: its principles and applications. W.W. Norton & Co.
Al-Khalili J. McFadden J (2015). Life on the edge: The coming age of quantum biology. New York; Crown.
Arndt M, Juffmann T, Vedral V (2009) Quantum physics meets biology. HFSP Journal 3:6, 386-400, DOI: 10.2976/1.3244985
Atasoy S, Donnelly I, Pearson J (2016). Human brain networks function in connectome-specific harmonic waves. Nature Communications 7:10340.
Ball, P (2011). Physics of life: The dawn of quantum biology. Nature 474: 272–274 (2011). DOI: doi.org/10.1038/474272a
Bigger JT Jr, Fleiss JL, Steinman RC, Rolnitzky LM, Kleiger RE, Rottman JN (1992). Frequency domain measures of heart period variability and mortality after myocardial infarction. Circulation Jan 85 (1):164-71
Blasone M, Jizba P, Vitiello G (2011). Quantum Field Theory and its Macroscopic Manifestations. London: Imperial College Press.
Bottaccioli F, Carosella A, Cardone R, Mambelli M, Cemin M, D’Errico MM, Ponzio E, Bottaccioli AG, Minelli A (2014). Brief Training of Psychoneuroendocrinoimmunology-Based Meditation (PNEIMED) Reduces Stress Symptom Ratings and Improves Control on Salivary Cortisol Secretion Under Basal and Stimulated Conditions. EXPLORE 10: 3, 170-179
Chiozza L (1998). Hidden effects in somatic disorders: psychoanalytic perspectives on asthma, psoriasis, diabetes, cerebrovascular disease, and other disorders. Psychosocial Press.
Cosic I (1994). Macromolecular bioactivity: is it resonant interaction between macromolecules? Theory and applications. IEEE Transactions on Biomedical Engineering 41, no. 12: 1101-1114.
Dal Lin C, Falanga M, De Lauro, E, De Martino S, Vitiello G (2020). Biochemical and biophysical mechanisms underlying the heart and the brain dialog. AIMS Biophys 8: 1–33.
Del Giudice E, Doglia S, Milani M., Vitiello G (1983). Spontaneous Symmetry Breakdown and Boson Condensation in Biology. Physics Letters A 95A- 9: 508-510.
Del Giudice E, Doglia S, Milani M, Vitiello G (1985). A quantum field theoretical approach to the collective behavior of biological systems. Nuclear Physics B 251 (FS 13): 375–400.
Del Giudice E, Doglia S, Milani M, Vitiello G (1986). Electromagnetic field and spontaneous symmetry breakdown in biological matter. Nucl. Phys B 275: 185–199.
Del Giudice E, Preparata G, Vitiello G (1988). Water as a Free Electric Dipole Laser. Phys Rev. Lett 61: 1085-1088.
Durickovic I (2016). Using Raman spectroscopy for characterization of aqueous media and quantification of species in aqueous solution. Applications of Molecular Spectroscopy to Current Research in the Chemical and Biological Sciences 405.
Freeman WJ, Vitiello G (2006). Nonlinear brain dynamics as macroscopic manifestation of underlying many-body dynamics. Phys of Life Reviews 3: 93 – 117.
Freeman WJ, Vitiello G (2016). Matter and mind are entangled in two streams of images guiding behavior and informing the subject through awareness. Mind Matter 14: 7-24.
Friston, K (2008). Hierarchical models in the brain. PLoS computational biology 4.11: e1000211.
Friston, K (2010). The free-energy principle: a unified brain theory? Nature reviews neuroscience 11: 2
Frohlich H (1968). Long-range coherence and energy storage in biological systems. Int J Quantum Chem 2:641-649.
Godlee F (2011). What is health? BMJ 343: d4817.
Goldbeter A (2018). Dissipative structures in biological systems: bistability, oscillations, spatial patterns and waves. Phil Trans R.Soc A 376: 20170376
Jadad AR, O’Grady L (2008). How should health be defined? BMJ 337: a2900
Kolesov BA (2021). Hydrogen Bonds: Raman Spectroscopic Study. International Journal of Molecular Sciences 22(10): 5380.
Havelka D, Cifra M, Kučera O, Pokorný J, Vrba J (2011). High-frequency electric field and radiation characteristics of cellular microtubule network. J Theor Biol 286: 31-40.
Huelga SF, Plenio MB (2013) Vibrations, quanta and biology. Contemporary Physics 54:4, 181-207, DOI: 10.1080/00405000.2013.829687
Lloyd S (2011). Quantum coherence in biological systems. J.Phys Conf Ser 302: 012037.
Marais A (2018). The future of quantum biology. J R Soc Interface 15: 20180640. DOI: 10.1098/rsif.2018.0640
Marty P (1980). L’Ordre psychosomatique. Payot, Paris.
Maturana HR (1995). Biology of self-consciousness. in: Consciousness: Distinction and Reflection. Ed. G. Tratteur (Naples: Bibliopolis): 145–175. https://cepa.info/639
McCraty R, Atkinson M, Tomasino D, Bradley RT (2009). The Coherent Heart: HeartBrain Interactions, Psychophysiological Coherence, and the Emergence of SystemWide Order. Integral Review.5, No 2.
McCraty R, Childre D (2010). Coherence: bridging personal, social, and global health. Altern Ther Health Med Jul-Aug 16(4):10-24.
McCraty R, Deyhle A, Childre D (2012) The global coherence initiative: creating a coherent planetary standing wave. Glob Adv Health Med 12 Mar;1(1):64-77.
McDougall J (1989). Theaters of the body: A psychoanalytic approach to psychosomatic illness. W.W. Norton & Co.
McFadden J, Al-Khalili J (2018) The origins of quantum biology. Proc R Soc A 474: 20180674. DOI: 10.1098/rspa.2018.0674
Messori C (2019). Deep into the Water: Exploring the Hydro-Electromagnetic and Quantum-Electrodynamic Properties of Interfacial Water in Living Systems. Open Access Library Journal 6: e5435.
O’Raifeartaigh L, Straumann N (2000). Gauge theory: Historical origins and some modern developments. Reviews of Modern Physics 72.1 (2000): 1.
Pattee H (1969). When does a Molecule become a Message? Communication in Development.
Pelling AE, Sehati S, Gralla EB, Valentine JS, Gimzewski JK (2004). Local nanomechanical motion of the cell wall of Saccharomyces cerevisiae. Science 305: 1147-1150.
Penrose R (1994). Shadows of the mind. Oxford University Press.
Persinger MA (1987). Geopsychology and geopsychopathology: Mental processes and disorders associated with geochemical and geophysical factors. Experientia 43, 92–104
Peskin M E (2018). An introduction to quantum field theory. CRC Press.
Plotkin HC (1994). The Nature of Knowledge: Concerning Adaptations, Instinct and the Evolution of Intelligence. London: Allen Lane, Penguin Press.
Preparata G (1995). QED coherence in Matter. World Scientific, Singapore.
Ricciardi LM, Umezawa H (2004). Brain physics and many-body problems. Kibernetik 4: 44 – 48, 1967. Reprinted in Brain and Being. Globus GG, Pribram KH, Vitiello G. Eds.; Amsterdam, Netherlands: John Benjamins Publ. Co: 255 – 266.
Salari V, Tuszynski J, Rahnama M, Bernroider G (2011). Plausibility of quantum coherent states in biological systems. Journal of Physics: Conference Series; Bristol 306: Fasc. 1, (Jul 2011). DOI: 10.1088/1742-6596/306/1/012075
Schützenberger AA (1998) The Ancestor Syndrome. Transgenerational Psychotherapy and the Hidden Links in the Family Tree. Routledge, Taylor & Francis Group.
Seager W (2009). A new idea of reality: Pauli on the unity of mind and matter. Recasting Reality: 83-97. Springer, Berlin, Heidelberg.
Sengupta B, Tozzi A, Cooray GK, Douglas PK, Friston KJ (2016) Towards a neuronal gauge theory. PLoS Biology 14.3: e1002400.
Thyrhaug E, Tempelaar R, Alcocer MJP, Zidek K, Bina D, Knoester J, Jansen TLC, Zigmantas D (2018). Identification and characterization of diverse coherences in the Fenna–Matthews–Olson complex. Nature Chem 10: 780–786.
Tozzi A, Peters JF (2016). A topological approach unveils system invariances and broken symmetries in the brain. Journal of Neuroscience Research 94.
Umezawa H (1993). Advanced field theory: Micro, macro, and thermal physics, New York, N.Y., USA: Am Inst of Phys.
Ventura C, Gullà D, Graves M, Bergonzoni A, Tassinari R, von Stietencron J (2017). Cell melodies: when sound speaks to stem cells. CellR4 5: e2331.
Vitiello G (1995). Dissipation and memory capacity in the quantum brain model. Int J Mod Phys B9: 973 – 989.
Vitiello G (2001). My double unveiled. Advances in Consciousness Research. John Benjamins Publishing Company.
Vitiello G (2004). The dissipative brain. In Globus GG, Pribram KH, Vitiello G. Eds. Brain and Being. At the boundary between science, philosophy, language and arts. Amsterdam, The Netherlands: John Benjamins Publ. Co: 315 – 334.
Vitiello G (2012). Fractals as macroscopic manifestation of squeezed coherent states and brain dynamics. Journal of Physics: Conference Series Vol. 380, No. 1: 012021. IOP Publishing.
Yagihara S, Kita R, Shinyashiki N, Saito H, Maruyama Y, Kawaguchi T, Tokita M (2019). Physical meanings of fractal behaviors of water in aqueous and biological systems with open-ended coaxial electrodes. Sensors 19(11): 2606.
